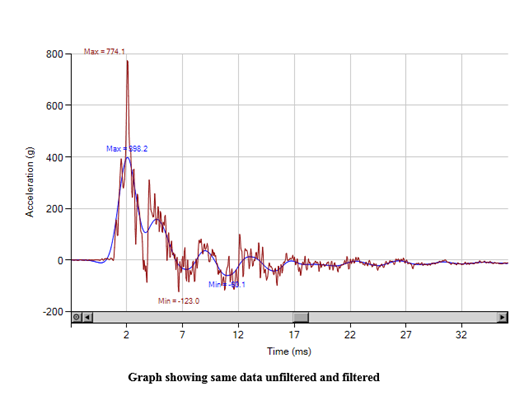
Introduction
This post presents C# code for a fourth order zero-phase shift low-pass Butterworth filter function. The code was converted to C# from code originally written in Visual Basic for Applications (VBA) by Sam Van Wassenbergh (University of Antwerp, 2007). I was using the VBA code for an application, and when I decided to convert the application to C#, I found there were not many resources for a Butterworth filter written in C#, so I converted the VBA code to C#, and hence I thought an article could be useful to others.
Using the Code
The function is shown below. The unfiltered data is passed to the function as a double[] array, along with the time step in seconds, and the desired cutoff frequency in Hz. The filtered data is returned, unless the input data indata is null, in which case, null is returned, or if the cutoff frequency is 0 then the original data is returned unfiltered. The time step variable deltaTimeinsec, which is the time between one data point and the next in seconds, is needed to calculate the sampling rate (inverse of the time step). The input data is prepared in a slightly larger array (4 extra points - two extra points at each end of the data), so that the real endpoints will have neighbors for the calculations. After the variables are assigned, the two loops perform the backward and forward traversal of the filter calculations. Then the output data is put back into an array the same size as the original for return.
public static double[] Butterworth(double[] indata, double deltaTimeinsec, double CutOff) {
if (indata == null) return null;
if (CutOff == 0) return indata;
double Samplingrate = 1 / deltaTimeinsec;
long dF2 = indata.Length - 1;
double[] Dat2 = new double[dF2 + 4];
double[] data = indata;
for (long r = 0; r < dF2; r++) {
Dat2[2 + r] = indata[r];
}
Dat2[1] = Dat2[0] = indata[0];
Dat2[dF2 + 3] = Dat2[dF2 + 2] = indata[dF2];
const double pi = 3.14159265358979;
double wc = Math.Tan(CutOff * pi / Samplingrate);
double k1 = 1.414213562 * wc;
double k2 = wc * wc;
double a = k2 / (1 + k1 + k2);
double b = 2 * a;
double c = a;
double k3 = b / k2;
double d = -2 * a + k3;
double e = 1 - (2 * a) - k3;
double[] DatYt = new double[dF2 + 4];
DatYt[1] = DatYt[0] = indata[0];
for (long s = 2; s < dF2 + 2; s++) {
DatYt[s] = a * Dat2[s] + b * Dat2[s - 1] + c * Dat2[s - 2]
+ d * DatYt[s - 1] + e * DatYt[s - 2];
}
DatYt[dF2 + 3] = DatYt[dF2 + 2] = DatYt[dF2 + 1];
double[] DatZt = new double[dF2 + 2];
DatZt[dF2] = DatYt[dF2 + 2];
DatZt[dF2 + 1] = DatYt[dF2 + 3];
for (long t = -dF2 + 1; t <= 0; t++) {
DatZt[-t] = a * DatYt[-t + 2] + b * DatYt[-t + 3] + c * DatYt[-t + 4]
+ d * DatZt[-t + 1] + e * DatZt[-t + 2];
}
for (long p = 0; p < dF2; p++) {
data[p] = DatZt[p];
}
return data;
}
Conclusion
A fourth order zero-phase shift low-pass Butterworth filter function was presented in the C# language as converted from Sam Van Wassenbergh’s original VBA code.
